Time Lapse

A 10-to-1 compressed video of Noel's Magic120Cell solution. Happy Holidays!

Magic120Cell Solved!

In a bit of a coincidence to the last post, the formidable permutation puzzle version of the 120 cell I published this year has been solved for the first time. Noel Chalmers posted this announcement to the 4D cubing group last night.  I have genuinely wondered if this might never happen, and as a result feel a strange urge to alert the press!

I am relieved the software held up to the task. His solution had over 33 thousand moves, which took about 40 minutes just to play back on my computer using the fastest move speed. It feels really good to have had a hypercubing enthusiast put that much effort into something I helped create.  A lot of love went into it earlier this year, and even as far back as 2006 when the hope of it was initially started, so it really is neat that an actual solution is now realized.

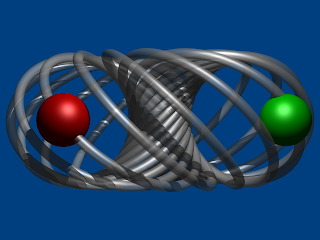
120 Cell Animations

Here are a couple quick videos of the 120 cell made for your enjoyment using 120 Cell Explorer. The animations show how the appearance of this object would change as we rotate our viewpoint around it in 4D.

Both show half of the 120 cells and color the cells based on which "ring" they are on. The highly symmetric 120 cell can be thought of as composed of 12 rings of 10 cells each, hence these animations are showing 6 of the 12 rings. One ring (the purple one) is more difficult to see because it is surrounded by the 5 others. All of the rings are linked to every other ring exactly once, so unless you were a magician, you couldn't pull any 2 of the rings apart without breaking one of them. Can you see the linking?

The next video is slightly more interesting to me. It is essentially identical to the first one except that we are starting from a different vantage point in 4D.

G3D Blog

I started a Gravitation3D blog this week.  Nope, I'm not masochistic and trying to burden myself with another project.  On the contrary, I did it after getting a flux of questions about the same time I read this article from a coding blog I like.  It took an hour or two to setup the new blog to a reasonable liking, but now every time I get a G3D question, I can answer it there instead of with an email (which would only shortly after be sentenced to a life term of solitude in my gmail).  I don't get a lot of emails about G3D, but over time this will probably make it even less so.  I figure this will bring me much closer to my lifelong goal of having no external sensory inputs impinge on my overly introverted self.

Why a wordpress blog this time instead of blogger?  I've discovered wordpress has support for LaTex, which allows nice display of mathematical formulas, and I think this will be important for some G3D posts.  There are roundabout ways to do this in blogger too (see here, here, or here), but I found this too troublesome - it didn't seem to work as well or look as nice as the native wordpress support.  

It's funny, since google gives you so much for free and at such high quality, I have found myself frustrated on more than one occasion when they haven't solved a problem for me that feels like it should be solved (like LaTex support for any html I publish).  Once I'm aware of this reaction, I realize it's a ridiculous sentiment, but I'd be lying if I said it doesn't crop up now and then (No good deeds go unpunished).

Hi, my name is Roice... and I crochet

Picture me and Sarah sitting side by side in bed around 11:30 pm, her knitting, me crocheting, and you'll have a pretty good idea of a number of evenings of ours over the last few weeks.  Maybe not the most exciting image, but we've been having fun.  I've finished my first crochet project now, a portion of a hyperbolic plane with radius of curvature of about 5 cm, and I think it turned out pretty good.  The crocheted rows are quite visible in this picture I snapped.  Are they geodesics of the surface? *

I'm sure I'll learn more by playing with it, but I've learned some already, not the least of which is that I can't count to 5.  All I had to do was 5 normal stitches for every doubled-up stitch, and I'd say 60% of the time I lost my place!  And boy are programmers spoiled with undo.  Too bad that functionality isn't available in the physical universe.

Something noteworthy about this particular construction (but not a property of hyperbolic geometry itself) has to do with the fact that the number of stitches in successive rows forms a geometric sequence, that is the length of each row is a constant multiple of the previous row.  That has some unintuitive side effects.  I did 23 rows total, the first had 20 stitches and took maybe a minute, but the last had over 1000 stitches and took almost 3 hours!  If I were to do another 23 rows, the final 46th row would take me over 28 days (no sleep, no breaks) and who knows how many skeins of yarn.  Add yet another 23, and the final row would take over 5 years.  This reminds me of "the magic of compounding interest", and what I've been told the value stocks are supposed to do in theory.

Speaking of economic unraveling, this leads to something else intriguing about my hyperbolic plane.  Instead of tying off the end when I was done, I could have undone the entire uber-knot in one fell swoop just by pulling out my crochet hook and gently pulling on the yarn.  It's like the whole thing is a house of cards, a deceivingly stable form that is actually no more substantial than the first slip knot that started the whole thing.  This reminds me of the axiomatic foundations of mathematics.

While working on this, I couldn't help but focus on a possible useful application.  I haven't figured it out yet, but my mind can't let go of the idea that this could solve the widespread problem of competition for blankets when couples sleep.  The extra material seems like a perfect candidate to provide some benefit here :)

* Nope.  If they were, I would be able to fold the surface so that they appeared flat and straight.

Sarah Goes Hyperbolic

Sarah has been knitting some pretty scarfs lately. She was showing off her latest project to me, and lo and behold it turned out to be mathematical! As she was knitting successive rows, she added incremental stitches to give it a ruffled appearance. I told her I thought this was especially cool because the extra stitches were giving the scarf a negative curvature. It was hyperbolic! That is what happens when you try to put extra material into what would otherwise be a flat 2 dimensional surface.  She lovingly rolled her eyes :)

Every time I think I have a new idea, it turns out someone has already been there, done that.  On the plus side, the article I then tracked down already contained developed information and instructions for crocheting your very own hyperbolic plane.  Following the directions will result in a hyperbolic surface of constant negative curvature (Sarah's scarfs don't adhere to the constant part).  I also found this site with some nice pictures of completed crochetings (the site mentions the work of Daina Taimina, who is one of the authors of the paper above).

It is interesting to note that if you build a constant negative curvature surface large enough, it will necessarily end up intersecting itself in our 3D world.  Models living in our physical universe are limited in their representation.  This is in contrast to models of constant positive curvature surfaces, which do fit nicely into the world.  The surface of any ball will do.

Sarah and I just returned from Hill Country Weavers, where Sarah bought me a crochet hook, so I'm now off to attempt creating my own hyperbolic plane!

update:  Sarah did not aprove the cuteness factor of my first picture, so I've uploaded an improved version.

Loxodromes!

This is a sweet word used to describe a sweet mathematical curve. And by sweet I mean awesome and one of my favorites. Speaking of favorites, I learned about these curves in my favorite mathematical book, Visual Complex Analysis, by Tristan Needham. If this post piques your interest, there is tons more about them there.

Loxodromes are curves of motion you get from certain kinds of Mobius transformations, whose general algebraic definition is the formula (az+b)/(cz+d).  z is a complex number here, that is a point in a plane. Hence, these transformations can be viewed visually by their effects on the points of a plane. However, Mobius transforms have an elegant interpretation when viewed as a corresponding transform obtained by unprojecting the plane onto a sphere (doing the reverse of stereographic projection that I described in a previous post), as the resulting motions on the sphere are much simpler! The sphere is called the Riemann Sphere in honor of Bernhard Riemann.

In this animation, I put a little white fuzzball near the north pole of the sphere to show the light generating the shadows. The shadows are the 3D->2D stereographic projection of the curve on the sphere. So this is two simultaneous views of a loxodrome, both on the sphere and on the plane (fine print: not exactly because the curve I've drawn has a little bit of thickness coming off the sphere surface, but the shadows are still close). If you remember the previous discussion in the soap bubble post about projecting from 4D->3D, hopefully that process is a little more clear by analogy now. We may not be able to look at 4D objects in our world, but we can look at their shadows! Anyway, I hope this shed a little more light (pun intended) on what stereographic projection is.

Loxodromes correspond to one of the more general types of Mobius transform motions, and the animation can help a little in explaining what I mean by that. Watch it for a bit and answer the following: Do you see the curve stretching and shrinking over the sphere like it is moving from one end of the spiral towards the other? Or do you see the curve as unchanging its shape and just rotating as a whole about an axis through the 2 spiral ends?

My perception is biased to the former, but there is no right answer because it could be viewed either way! The first kind of motion is called hyperbolic (unfortunately, the meaning here is not the same as of the last post), and the second is called elliptic. Both are special types of Mobius transformations, and loxodromes are what you get when you do both in combination. Incidentally, it is interesting that I have trouble seeing this as a rotating curve because that is how the POV-Ray script actually generated the sequence of images :)


Recognize the projected shape in this picture? A special case of the projection of a loxodrome onto the plane is a logarithmic, or equiangular spiral! Yes, the spiral of sea shells and galaxies and so much more. Are you feeling the awesomeness yet? By the way, I took advantage of this to simplify making the movie. There are no complex number calculations explicitly going on, just a function that can generate points of a logarithmic spiral on a plane, and a function that can unproject those points from the plane to a sphere. So if you were thinking it was terribly involved to generate the movie and picture, it actually was not so bad (though it did take a long time to render out the frames). The entire definition file is only about 100 lines, with half of it standard required stuff (camera position, etc.). Here it is if you care to check it out.  POV-Ray is great! 

Hyperbolic Tiling in Motion

I've seen a number of pretty images of hyperbolic tessellations (tilings) on the web. A nice gallery from one of the producers of the Dimensions videos turns many flat Escher tilings into hyperbolic ones. What I hadn't seen until I went looking the other day was a way to animate motions of the hyperbolic plane (using the Poincare Disk model).

I thought this would be a cool project, and decided to do a quick search to see what applets out there might already be doing it. I was pleased to find this one right at the top of the search results (and even more pleased because I know the author Don Hatch through the Rubik hypercube group and was able to meet him some time ago...wow actually that was almost a decade ago...scary).

Drag your mouse around on his applet above to see this tiling of the hyperbolic plane translate (update: Sarah let me know this doesn't work in Google Reader btw). This can give a much better feel than a static picture that each tile in the image is actually the same shape (a regular polygon). I know this post is lacking in background, but hyperbolic geometry can't be represented through normal plane geometry without distortion, hence there are alternate representations with tradeoffs in characteristics. One thing to notice is that the white tiles have 7 sides and the dual blue tiling has 7 triangles meeting around a point, but you can't have such a tiling in normal space with a set of regular triangles (7*60>360). There just isn't enough space, kinda like our closet storage for Sarah's shoes :)

This applet is highly configurable and fun to play with, e.g. click on it and start pressing p. You can find all the info on Don's applet page. There are some extensions I would still like to see in addition to translations. A rotation of the plane about any selected point would be cool. Hyperbolic geometry is more interesting than flat (Euclidean) geometry, leading to more possibilities as well. Specifically there is a special kind of rotation called a limit rotation, and this would also be neat to see. Finally, it would be sweet to allow animated motions of other models of hyperbolic geometry like the half plane model. So there is still fun potential hobby coding to be done (of course).

World of Goo

Against one of the stereotypical traits of those practicing my chosen profession, strangely I have never been much of a gamer, at least not since junior high school. In truth, I haven't bought a video game in years, but last weekend I did! (And it wasn't even a racing game.) It is a physics game, but you might never realize it. The art and music are both extremely cool, and there is one song I wish I had in my iTunes library. Here is a review that will do it more justice than I could.

They have a free demo, which was more than enough for me to want to support these two guys, who are only asking $20 for the full version as well...excellent joy-to-price ratio. The main site is here, which has some nice trailers if you'd like to see more before downloading the demo. Thanks Rob for pointing me to this one :)

Soap Bubbles

I've been playing around with POV-Ray in the background quite a bit since my first post about it. I spent a lot of evening hours hanging out with Sarah in front of the tv working on a soap bubble effect (I had no idea this would turn into the diversion it did), and I wanted to share my best effort on it so far :)

(click for a larger version)

It took some obsessiveness to get this far. Sarah was helpful, and after a number of iterations I'm sure she tired of being shown sets of two ever-so-slightly-different pictures to choose the best one. Luckily she humors me.

I want to share a little more about this picture because I wasn't thinking about rendering soap bubbles at all when starting it. That really was just a diversion. I am interested in projections from 4D to 3D of the hypersphere (the four dimensional mathematical analogue of a familiar sphere) because I am trying to understand that object better. Just like a sphere in 3D, this is the set of points that is a fixed distance from a center point, only in a higher space. (I feel the need to give some background - an ordinary sphere is a surface of dimension 2 that lives in 3 dimensions.  A hypersphere is a 3 dimensional object, and mathematicians call these the 2-sphere and 3-sphere, respectively - the names can lead to confusion because in an everyday sense most think of a sphere as a 3D object rather than a 2D one.  Spheres don't even have to be embedded in a higher dimensional space, but that is a digression here.)

I'm going to continue to blab a bit, but stay with me (or just skim this paragraph) because the most interesting part I want to share is at the end of this post...  We can't stereographically project every point of the hypersphere from 4D down to 3D (the resulting set of points would cover all of 3D space plus one more point at infinity, and hence the full projection doesn't help my unfailing need to see pictures of things to understand them). So we try to do the best we can by looking at discrete subsets of points (and lines, and surfaces) of the hypersphere. The picture above is one such subset, a regular tiling of the hypersphere called an 8-cell, and is more commonly known as the hypercube. This is one way of representing the hypercube anyway, which involves first stretching the edges of the 4D cube outwards so they lie on the hypersphere (causing them to be curved), then stereographically projecting that from 4D to 3D. I'll (hopefully) explain more about stereographic projection when I share a short animation I've been working on in POV-Ray (if you're too excited to hold your breath for that, check out dimensions-math.org for some excellent and free downloadable videos. I recommend those regardless!!).  Some more cool properties about the picture above... All the edges are "great circles" of the hypersphere, and all the bubble surfaces are "great spheres" of the hypersphere. Stereographic projection preserves circles and spheres, meaning a shape that is a circle in 4-space before the projection is still a circle after the projection. How cool is that? (Before you go on an extrapolation binge like I always do, know that the centers of the circles and spheres are not preserved during projection though, i.e. the center of a projected circle is not the projection of the center of the corresponding unprojected circle.  Peter piper picked a peck of pickled peppers.)

No worries if the above didn't make too much sense, but let me share something really astonishing about all this. Amazingly, you can make the shape of a hypercube like this picture in our real 3D universe with real bubbles because of the way bubbles try to minimize their surface area! Here is a youtube video showing a bubble performer make a "square bubble". But taking the above into consideration, the performance is really is much more spectacular than a square bubble. Using only bubbles, he's effectively projected a 4D object (a regular polychoron) for us to see and admire! This is truly magical and a nice example of the unreasonable effectiveness of mathematics in describing our physical universe.

We can theoretically "project" more complicated tilings of the hypersphere using bubbles as well. Though I don't imagine a bubble magician could pull this one off, here is a computer rendering of the 120-cell in bubbles.

Couple all this with Sarah discovering it is tons of fun to blow bubbles off our balcony, and there are smiles all around :)

(btw, sorry for all the parentheses (I can't seem to help myself (it's what I do)))

Between the Folds

Sarah and I watched a neat documentary blending art and mathematics on Thursday night. There are many interesting and endearing characters in this story, and we both really liked it. It is an hour long and you can watch it online, but only this weekend as part of the Hamptons International Film Festival. If you don't catch it there, I recommend bookmarking the film site so you can see it at some point (they have a trailer at that link btw).

Obey Gravity. It's the Law.

What do you get when you start out with a vertical line of masses and set them in motion around a heavy object?

beauty!

I've been working on allowing screen captures of my programs this week, which I can package into video with the opensource ffmpeg, and I am really liking it.  This Gravitation3D system (admittedly an artificial configuration) is very similar to the one I used to to make the pic on the top of my blog.

$300 Unicycle Trumps $60000 Lotus

This happened a while ago, but the story recently came up in conversation and is maybe worth a funny share. For a long time, there was this fancy Lotus dealership just a few blocks down the street from where I work. These cars are pretty wild looking and come in some crazy colors...definitely eye turners.


On one particular morning when I was peddling into work on my unicycle, I had the serendipity to ride past one of these Loti driving down the street in the opposite direction. And I wish I could have videotaped the slowed driving and jaw dropped, head following reaction of the driver as we cruised on by each other. I'm telling you (somewhat seriously)...if you're having a midlife crisis and want people to look at you, meet that need for 0.5% of the cost of the traditional approach by learning to ride a unicycle!

Anyway, my friend Aj has a yearly ride in San Antonio for his birthday. Last year there were 11 riders, but last Sunday we reached critical mass with 18. I like riding in the big groups because I'm not much of a ham, so it feels like I'm getting to ride incognito for a change. It seems the only good camouflage for a unicyclist is more unicyclists. Here are some photos from the ride...

Small Talk

I really like riding the bus to work because it is my time to read. Sometimes though there are forces at work conspiring to steal these precious moments from me. For some reason, a number of bus travelers looooove to hear themselves talk, and even after a year of bus riding I haven't yet mastered the fine art of evading this with advanced techniques such as using ear buds or avoiding eye contact. Yesterday, I found myself daydreaming about other approaches, even extreme ones like feigning deafness, while my morning commute was usurped by a nice, but chatty man who talked to me ("to" is deliberate here, as to be distinguished from "with"). I got on the bus with another 4x4x4 cube for Colby (the first sadly has been disassembled and irreversibly mixed with food, but that is another story), and with this ice breaker the "conversation" began...

Nice, Chatty Man: "Oh, a Rubik's cube! I used to be able to solve those."

Me: "Cool, this one is for my nephew Colby."

Nice, Chatty Man: Proceeds to talk uninterrupted for 25 minutes with absolutely no peep from me as I longingly make glances at my book - I'm in no way exaggerating. Rubik led to speed solving, to games in general, to ping pong, to his forte: billiards. There's straight pool, snooker, a rotation game, 9-ball, some variant using 2 cue balls (one having a red dot), big and little tables and pockets, english and masse shots. He had what he called a "senior moment" at one point trying to remember the rules of one of the multitude of games he was describing to me, and pointed out this happens to everybody. Gambling came up, and the high-rollers he's played with. Snooker is actually named after a fish, which has really sharp teeth and hence requires one to use steel lead lines when fishing. There was in fact extended explanation of fishing, though I can't recall the details (it happens to everybody), with occasional reversals in the exposition back to pool again. Jackie Gleason made a cameo in this story and he described their meeting and how Jackie offered him Dom Perignon while they played (aside: nice, chatty man has actually had many tens of gallons of this fine sparkling wine over the course of his lifetime). A random glance out the window and a '65 something or other caught his attention, so we were off to the world of cars, 352s, hemys and chevys, oh my..........

Me: "Well, this is my stop, seeya."

Nice, Chatty Man: "Very nice talking with you. Bye."

Truly he had a dizzying intellect. I feel guilty for feeling a bit miserable during this experience and in retrospect, maybe I should have just tried to help get this guy setup to do some blogging.  After all, I didn't know snooker was both a game and a fish, nor what a masse shot was called.  But I can't even begin to decide how to tag this one.

Colby Cade Conquers the Cube!

My nephew Colby dramatically surprised me last Thursday when Melanie posted a video on the Wright's blog of him solving a Rubik's Cube! It totally made my day, and I'm posting it here as well :)

I am compelled to fan the flames of this fire and so I immediately went and bought him a 4x4x4 (hopefully this is not a bad thing). Since they are in Austin due to Hurricane Ike, I got to surprise him with it sooner than expected. Here he is in action last night with the new challenge.


At some point I'll need to decide if I should warn that continuing to increment the number-per-side on your cubes is ultimately a losing proposition.

We Can Fly!

Sarah and I spent Sunday taking an introductory hang gliding class with our friends Tim, Amir, and Sarah (Robbins - we try to maximize the number of Sarah's in our life). It was very exciting, and I am very sore today. We took our class from Jeff Hunt.

Sarah had this sweet sail on her 4th run down the hill.

I never landed as nicely as she did there :) ...which is probably why I'm so sore. Here are more photos we took.

Sarah and Amir took a bunch more nice photos as well.

Looking Beautiful

Sarah is on a mission to keep me looking young. She has developed quite an intense program for me. Specifically, witness this photo I snapped...


You might not be able to read all the labels she did on there, but there are various instructions about even/odd days (she is good at appealing to my numerical sense), whether to use in the morning, evening, or both, etc.

Now, I'm willing to try just about anything at least once (dare I admit I've had a pedicure?), so I stuck with it for a bit. The results were actually quite surprising to me, and even to Sarah. Here are some comparison photos. Before...

Notice the crows feet around the eyes, forehead wrinkles, generally unhealthy skin. After only two weeks, voilà!

Now I just have to keep sticking with it!

Mathematical Education

Yesterday I finished reading "The Poincare Conjecture" by Donald O'Shea. It was an enjoyable book with lots of interesting information. He comments near the close:

"It is up to all of us to ensure that the legacy of our times is a society that stewards and develops our common mathematical inheritance. For mathematics is one of the quintessentially human activities that makes us more fully human and, in so doing, leads us to transcend ourselves."

Earlier O'Shea had also referenced an essay by William Thurston titled "Mathematical Education" that I found great. The following comment regarding curriculum centered around standardized tests was especially entertaining.

"We don’t diagnose pneumonia with only a thermometer, and we don’t attempt to cure it by putting ice in a patient’s mouth. We should take a similarly enlightened attitude toward testing in mathematics education."

It makes me feel sad that there is not more general interest in mathematics, and that the concept of mathematics developed in many minds is a perceived equivalence with rote arithmetic. But to finish on a positive note, there is amazing math being done out there. And even for an amateur, there are an incredible number of avenues and groups to pursue this lovely, transcending activity.

First POV-Ray Experience

Surprise, surprise, I am already falling behind on this blogging experiment.

I started playing with POV-Ray, which is a free ray tracing program. It quickly exceeded my expectations of quality and ease of use - really amazing in my opinion. Here are a couple of my first learning renderings, all of which have surprisingly short definition files.

I also made a short POV-Ray animation, so this turned out to be the reason for my first use of YouTube as well...

I'm not a vegetarian because I love animals. I'm a vegetarian because I hate plants!

I can't think of a better death sentence for all plants than to be digested alive!

In truth, that was just a funny bumper sticker I saw once. Sarah and I haven't been vegetarians, but we are giving it a shot for one month to see how it feels. Over ten years ago, I remember saying I'd "consider" (in quotes because I don't think I really did) being vegetarian for health reasons and that the benefit for animals was simply a side bonus. Today, I think that sentiment is reversed. Yay health, but humane treatment of animals is what I think might be able to get me to actually do this. I've been considering trying for a few months now, ever since I read Douglass Hofstadter's I am a Strange Loop, which has some inspiring discussion on the topic. It is a book about consciousness (the consciousness of animals relevant to talking about how we treat them).

And it does feel like it will be difficult, hence starting out small with a one month goal. I'd be lying if I didn't admit I love Rudy's BBQ. Hopefully the difficulty will only be at first and become easier over time. That is exactly how it went with sodas when we challenged ourselves to quit them for a year. Now I wonder how I used to drink them instead of wondering how I could live without them. Maybe by posting publicly about this (sorta publicly, since no one is reading), guilt can drive this endeavor beyond a month, but with any luck maybe it will just end up feeling better both for body and mind and no guilt will ultimately be required. Or maybe the satisfaction of violent plant deaths... Anyway, I'll let ya know how it goes.

7x7x7

I was excited to get a 7x7x7 puzzle I ordered from http://www.v-cubes.com/ in the mail this morning!!

It didn't stay in perfect harmony for very long, as my lovely entropy generator Sarah was quick to permute it into a dazzling disco ball of colors.

Hopefully I'll be able to put the universe back in order soon. If your inner chi is feeling off-kilter for a while, you'll know I'm still struggling with it...

Obsessive Email Editing

I never just sit down and write an email. Often the process starts with an outline, followed by a first draft, 20 rereads of word edits/grammar changes/structure reworks, a final publication candidate, and ultimately only concludes after an extended period of hanging over the send button in a paralyzed state. Sometimes this behavior is more overtly ridiculous, as when I need only produce a quick response to a friend, but rewrite the single sentence half a dozen times, investigating all subtleties and nuances of every possible word choice and sentence permutation, until I don't even know what sounds right anymore. My silly justification - I wouldn't want them to take my nice intentions even minusculely negative on any level whatsoever! In these situations, when I pass my pre-print over to my editor Sarah for the final ok, she rightfully refuses to even look at it. In other instances where a review might actually have a small iota of justification, she doesn't necessarily play the editor role (though she does that for me sometimes too), but instead often opts for the role of pressing the send button before I have a chance to stop her. I'm so thankful she's not like me sometimes :)

Naturally, this behavior will show itself in any writing I do, so maybe this blogging thing is going to be bad for me? (yes, I of course tortured myself with this post)

Ignorance is Bliss (or is it?)

"Behold yon miserable creature. That Point is a Being like ourselves, but confined to the non-dimensional Gulf. He is himself his own World, his own Universe; of any other than himself he can form no conception; he knows not Length, nor Breadth, nor Height, for he has had no experience of them; he has no cognizance even of the number Two; nor has he a thought of Plurality; for he is himself his One and All, being really Nothing. Yet mark his perfect self-contentment, and hence learn this lesson, that to be self-contented is to be vile and ignorant, and that to aspire is better than to be blindly and impotently happy. Now Listen."

- Edwin Abbott, Flatland

Turtles All The Way Down

I recently had the thought of doing a post on infinite regress. In fact, I felt I had stumbled upon the perfect idea to describe exactly what it was. After a little web searching, as luck would have it I found that this notion was already in another blog post. It was such a fantastic exposition of infinite regress in fact, that if the concept is fuzzy to you now, after studying that post a little things will become crystal clear. I can not emphasize enough that you must immediately go there and read it, starting at the top.

By the way, if you followed my advice and still made it this far, I guess that is the difference between a computer and a human. Somehow humans have learned to do a decent job avoiding the trap of the halting problem in most circumstances.

Blog Induced Trippy Self Reflection

I started a blog today. I've been resisting the urge for some time because I know with very high probability that I will get in only a few entries before it falls to the wayside, then all will be lost to cyperspace and the bots and if I'm lucky, someone else looking for blogspot names in 5-10 years (I'm not a pessimist, I'm a realist!). But most of my siblings have them, my mom has one, my dad has effectively had one since before there was such a thing, my friend's kids now have them, the neighbor's cat does, etc. etc. etc. and so the urge must be quelled. At least the future web surfer won't be cursing that I stole the best blog name. This happened a dozen times to me while searching for cool blog names that turned out to be taken from people who did what I'm about to do, but back in 2001. How dare they waste such unique blogspot names with so little content!! Truthfully, none of those names turned out to be right anyway.

Naming a blog is impossible, both the web link and the blog title. After giving up on a creative link because they were all taken, I settled on my standard roice3. For the title, I was going to do better and brainstormed "digressions of a dodecadork", which is somewhat apropos. But I felt too insecure to brave that one after Sarah text messaged me "Wow that may be the nerdiest thing I've ever heard." Besides, I'm not a dork anyway (though I am a nerd - you may be surprised to find out there is a difference...oh wait, maybe knowing that means I am a dork). Anyway, I acquiesced to my lack of wit by entering the title "roice". After staring at it for like 5 minutes trying to think of something better, I had a very weird, almost out of body, experience. This short label that is supposed to embody me suddenly looked terribly foreign and strange. The characters appeared as abstract symbols, and I had images of what "roice" might mean to my friends, siblings, and cyberfriends. Through all these eyes I suddenly felt as if I was losing grasp of the natural feeling of my own name. Since the idea of this blog is similarly ill formed, clearly this meant I was already sitting on the right title, and bonus, the experience will now get to be the subject of my first post. You should definitely try this interesting experiment future web wanderer. Write your name down and contemplate it in a self-reflecting manner until it freaks you out. Maybe it would even be better doing this in front of a mirror (I didn't try this).